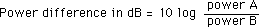
The decibel (abbreviated dB) must be the most misunderstood measurement since the cubit. Although the term decibel always means the same thing, decibels may be calculated in several ways, and there are many confusing explanations of what they are.
The decibel is not a unit in the sense that a foot or a dyne is. Dynes and feet are defined quantities of force and distance. (You can go to the National Bureau of Standards and look at a foot or a dyne if you want to. They never change.) A decibel is a RELATIONSHIP between two values of POWER.
Decibels are designed for talking about numbers of greatly different magnitude, such as 23 vs. 4,700,000,000,000. With such vast differences between the numbers, the most difficult problem is getting the number of zeros right. We could use scientific notation, but a comparison between 2.3 X 10 and 4.7 X 10 to the 12th is still awkward. For convenience, we find the RATIO between the two numbers and convert that into a logarithm. This gives a number like 11.3. As long as we are going for simplicity, we might as well get rid of the decimal, so we multiply the number times ten. If we measured one value as 23 hp and another as 4.7 trillon hp, we say that one is 113dB greater than the other.
The usefulness of all this becomes becomes apparent when we think about how the ear perceives loudness. First of all, the ear is very sensitive. The softest audible sound has a power of about 0.000000000001 watt/sq. meter and the threshold of pain is around 1 watt/sq. meter, giving a total range of 120dB. In the second place, our judgment of relative levels of loudness is somewhat logarithmic. If a sound has 10 times the power of a reference (10dB) we hear it as twice as loud. If we merely double the power (3dB), the difference will be just noticeable.
[The calculations for the dB relationships I just gave go like this; for a 10 to one relationship, the log of 10 is 1, and ten times 1 is 10. For the 2 to one relationship, the log of 2 is 0.3, and 10 times that is 3. Incidentally, if the ratio goes the other way, with the measured value less than the reference, we get a negative dB value, because the log of 1/10 is -1.]
Remember that the dB is used to describe relationships of POWER. Power is not often conveniently measured, especially in electronic devices. Most often we measure voltage and use the formula P=E squared over R to get power. Squaring a value doubles its logarithm, so our dB formula becomes:
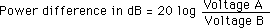
Power of sound varies as the square of pressure, so this formula is also appropriate for SPL (sound pressure level) calculations.
The final confusion comes from the concept of RELATIVE power. The question "relative to what?" has no single answer. The standard level (0dB) is chosen to be some convenient value for the application. For acoustics, 0dB often means the threshold of hearing, 0.0002ubar (Microbars: a bar is the "normal" pressure of air). Acousticians deal with positive values and call their measurements dB SPL. Electrical engineers use several meanings for 0dB. They sometimes remember to add a letter to the dB symbol to indicate which is intended.
0 dBj = 1 millivolt
0 dBk = 1 killowatt
0 dBm = 1 milliwatt at 600 ohms
0 dBv = 1 volt
There are many more. The power calculations must also take spectrum into account: it is not valid to compare a noise signal to a sine wave without some correction factor. The simple rule is to always compare similar signals.
The reference encountered most often in electronic music is 0 dBVU. dBVU are calculated just like dB with some extra restrictions on bandwidth and ballistics of the meter used. The VU (or Volume Unit) system is a hangover from early radio usage when 0 VU meant 100% of the legal modulation for the particular radio station. The level meters were all marked with percentage numbers as well as dBVU, and the numbers above 0 were in red. When tape recorders were invented, the same meters were used, and 0 dBVU came to mean the recommended operating point for the tape in use. The tape manufacturer supplied calibration tapes, and the machines were adjusted to give a 0 dBVU reading on the meter when those tapes were played.
0 dBVU on tape recorders has been creeping up over the years.The old Ampex standard was 185 nanoWebers/meter (a measurement of magnetic field on the tape), the most common today is 250 nW/m, and people are talking of the advantages of 500 nW/m. The cassette standard is 160 nW/m.
0 VU is not the maximum allowable signal on analog tape recorders. Most tape decks will cope with +6 or even +15 for brief times (such levels might damage the VU meters if sustained) and other devices will go up to +25. Any operating area above 0 VU is called the headroom.
0 VU is the maximum allowable signal on digital tape recorders. Exceeding that level will usually cause gross distortion in such devices.
The minimum useful signal is limited by the level of the ever present system noise. This is the NOISE FLOOR, and may be as high as -40 VU on a cassette deck or as low as -100 VU on a digital recorder.
Peter Elsea 1996