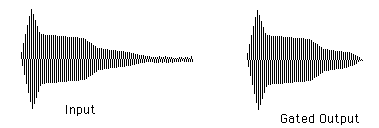
The devices collectively known as audio processors were originally added to the recording studio to allow compensation for frequency response or dynamic range problems in the equipment. When carefully used, they can add to the fidelity of the sound, and occasionally improve on reality. If improperly used, they can seriously degrade the sound or even produce laughable results. It is possible to use audio processors to manipulate sounds into unrecognizablilty, so naturally they are popular with composers of electronic music.
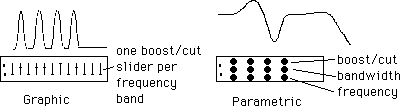
An equalizer is a device that can alter the spectral content of a signal. This can be done with any circuit that has an adjustable frequency response, the most familiar being the tone controls on a home stereo set. These tone controls typically affect the amplitude in two frequency regions, the treble and bass. This is sufficient for the minor changes the end user may wish to make in the program, but the recording engineer needs more flexibility and coverage of the entire audio spectrum. The complete studio will have complex e.q. systems that might include 30 or more regions of control. Many of these machines have sliders to adjust the amplitude of each band, and those sliders are laid out in such a way that their positions visually indicate the frequency response. This feature gives rise to the name GRAPHIC EQUALIZER.
A 30 band equalizer is going to be an expensive device, simply because of the sheer duplication of circuitry. Much of this circuitry is wasted most of the time because typical use only involves adjustment to two or three bands while the others are left alone. An equalizer with bands of adjustable frequency is a more economical approach to the problem, because only three to five circuits are necessary. Such an equalizer is called a PARAMETRIC EQUALIZER. Parametric equalizers typically have three controls for each band, allowing adjustment of frequency, amplitude, and width of the band.

Fig. 1. Equalizers
Equalizers are deliberately designed to create fairly minor changes in the signal. For more drastic effects, such as removing some region of the signal entirely, a FILTER is required. A filter is a circuit that sharply reduces the amplitude of signals of frequency outside of specified limits. The unaffected region is called the PASSBAND, and the filter type is named after the passband as low-pass, high-pass, or band-pass. The point where the signal attenuation just becomes noticeable (a reduction of 3 dB) is termed the CUTOFF FREQUENCY. A low-pass filter with a cutoff of 500 hz will attenuate any signal of frequency above 500hz.
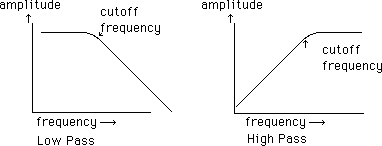
Fig. 2 Filter response curves
The attenuation provided by a filter is never absolute. A graph of the frequency response of the filter shows that the amplitude of a signal decreases as the frequency moves beyond the cutoff. The actual rate of this decrease is a parameter of filter design called the SLOPE. For arcane reasons the slope is always some multiple of 6 dB of attenuation per octave of signal frequency change. (A 6dB per octave filter is really not much more than an equalizer, and the drastic effects used in electronic music generally require 24 dB per octave circuits.) The slope is a general description of response at some remove from the cutoff. Various circuits differ in the shape of the curve near cutoff, and the possible shapes have names such as Bessel and Chebychev, after the originators of the math formulas involved.
Another design parameter which affects the shape of the filter curve is known as "Q". The derivation of Q is too complex for this discussion, but it is useful to know that filters with a high value of Q have an amplitude bump near the cutoff frequency and have a tendency to oscillate at that frequency.
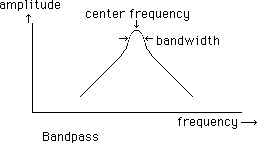
Fig. 3 Bandpass response curve
Bandpass filters have two cutoff frequencies. The difference between these frequencies is the BANDWIDTH, and the mean of the two is the CENTER FREQUENCY. Bandpass filters are sometimes encountered in large groups of fixed frequency circuits similar to graphic equalizers. These filter banks are often called 1/2 octave or 1/3 octave filters after the spacing of the filter bands. Such devices used to be the mainstay of many tape music studios before stable synthesizer filters and parametric equalizers were available. The characteristic pitches associated with those machines is almost a trademark of early 60's tape music.
The 1/3 octave filter was originally designed as a research tool for use in the spectral analysis of sounds. The amplitude of the output of each filter band can be separately measured and those measurements plotted on a graph to show the spectral content of the signal. New versions of the spectral analyzer generate data suitable for feeding directly to a computer for measuring complex time/spectrum relationships.
The most complex filter system around is the VOCODER. This device contains a filter bank set up for spectral analysis of a signal and a similar filter bank set up to process a second signal. The measurements derived from each filter in the analysis bank are used to control the amplitude of the signal from the corresponding filter in the processing bank. This system will impose the spectral shape of the analyzed signal onto the processed signal. The effect produced is quite striking, especially if voice is used as the sound for analysis.
It is a very common requirement of electronic devices that the amplitude of the input signal must not exceed some value. The typical result of failure to obey that rule is extreme distortion and possibly damaged speakers. If the operator of an audio system cannot be trusted to keep the signals within bounds, it is necessary to include a LIMITER, a device which turns the gain down whenever the signal exceeds the limit.
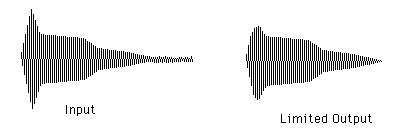
The action of reducing the amplitude of a signal can easily become a form of distortion, so the design of limiters is a delicate art. The usual approach is to set up the circuit so the transition from free to limited operation is somewhat gradual, and so that the transition back (called the RELEASE) is even more gradual. Appropriate release times vary with the nature of the program material, so this parameter is often adjustable. The limiting point (called the THRESHOLD) is of course also adjustable.
It is also possible for the soft spots of a program to become too low in amplitude, especially in a noisy medium like AM radio. A program with a wide dynamic range may have to have that dynamic range reduced in order to fit a particular audio system. (Surprisingly, the current switch to digital audio is making this problem more common as stations try to fit the wide range of compact discs through the restricted dynamic window of FM transmitters.) A device which reduces the overall dynamic range of a signal is called a COMPRESSOR.
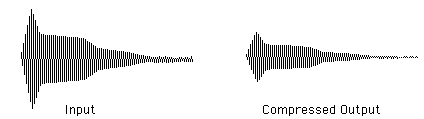
A compressor works by measuring the average amplitude of the input signal and using the information from that measurement to control the gain of an amplifier. If the measurement comes out above an adjustable threshold, the gain is reduced, if the measured input amplitude is below the threshold, the gain is increased. The amount of effect is usually adjustable and is expressed as a ratio of input dynamic range to output dynamic range, such as 2 to 1 or 3 to 1.
The release time is adjustable just as with the limiter, but the adjustment is much more critical, because the compressor is constantly changing gain whereas the limiter only cuts in occasionally. It is impossible to find a release time that is perfect for all situations. A very short release time will cause the circuit to attempt to trace the waveform of low frequency components of the signal, resulting in intermodulation distortion of high frequency components; whereas a long release time will leave the amplifier in the wrong mode if the signal changes quickly. That second error results in brief bursts of expansion, a very disconcerting effect. (Sounds like heavy breathing.)
Expansion is the opposite of compression. It is accomplished with the same circuit as compression, but the rules are changed so that any signal with amplitude above the threshold will be amplified and any signal with amplitude lower than the threshold will be attenuated. Expansion is used primarily for special effects, such as super loud tacos.
Sometimes a program is encountered which has proper dynamic
range but which has objectionable background noise. Very little
can be done to reduce the noise without affecting the signal,
but the spaces where there is no signal can often be cleaned up
with a NOISE GATE. This circuit is an expander that only works
on the low amplitude parts of the signal. Any section that is
below the threshold will be attenuated rather drastically, so
the space becomes nice and quiet. You can hear this device in
action on old movies on TV. Release time adjustment is very critical
in this application also, because if the circuit is too slow,
the turning down of the noise will be painfully obvious, but if
the circuit works too fast, the reverb at the ends of the sounds
will be lost.